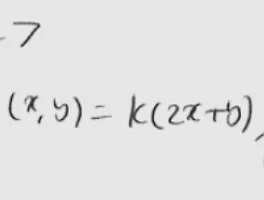Check
- Poisson distribution의 RV는?
- Poisson distribution의 식은? 평균과 분산은?
- Exponential distribution의 RV는?
- Exponential distribution의 식은? 평균과 분산은?
- 두 분포의 관계는?
- K-order erlang distribution이란? Exponential distribution과의 관계는?
- Erlang distribution의 평균은?
- X가 a~b일 때, uniform distribution의 평균과 분산은?
- 데시벨이란 무엇인가?

Poisson distribution과 exponential distribution의 간단한 복습과, exponential distribution의 forgetfulness의 증명이다.

Poisson distribution에서 P(X>=1)은 t 안에 event가 1번 이상 발생할 확률이고, 이것은 다시 말해 첫 event가 발생하는 시간 간격이 t보다 작을 확률이다 -> 의미적으로 exponential distribution의 cdf에 t를 넣은 것과 같다. 따라서 poisson의 확률 분포를 이용해서 P(X>=1)을 구하면 exponential distribution의 cdf를 유도할 수 있다.

Poisson과 exponential의 관계에 대해 추가적으로 조사한 내용이다. 어떤 사건의 발생율을 공통된 parameter로 가지고, poisson은 단위 시간당 발생 횟수를 모델링하고, exponential은 그 사건의 발생 간격을 모델링한다고 할 수 있다.

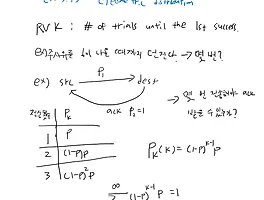
1차 erlang distribution = exponential distribution이다. K개의 시간 간격의 합을 RV로 가진다.

기대값의 유도는 위와 같다. 분산의 유도는 생략한다.

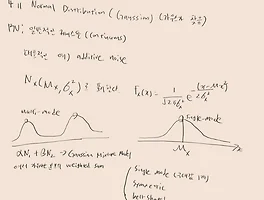
Uniform distribution의 평균과 분산, pdf이다. Quantization error를 통해 uniform distribution이 실제로 사용되는 사례를 보였다. Delta 값이 올라갈수록 error의 분산이 올라가서 신호가 불안정해진다. 때문에 delta를 dB를 척도로 하여 조절한다.
'수업 정리(개인용) > 확률 및 통계(한양대 이상화교수님)' 카테고리의 다른 글
| 확률 및 통계(한양대 이상화교수님) lec10 : 다중변수 및 연합분포 (0) | 2021.02.01 |
|---|---|
| 확률 및 통계(한양대 이상화교수님) lec9 : 정규분포 (0) | 2021.02.01 |
| 확률 및 통계(한양대 이상화교수님) lec7 : 여러가지 이산확률분포 (0) | 2021.01.20 |
| 확률 및 통계(한양대 이상화교수님) lec6 : 조건부 평균 (0) | 2021.01.12 |
| 확률 및 통계(한양대 이상화교수님) lec5 : 확률변수의 평균과 분산 (0) | 2021.01.11 |